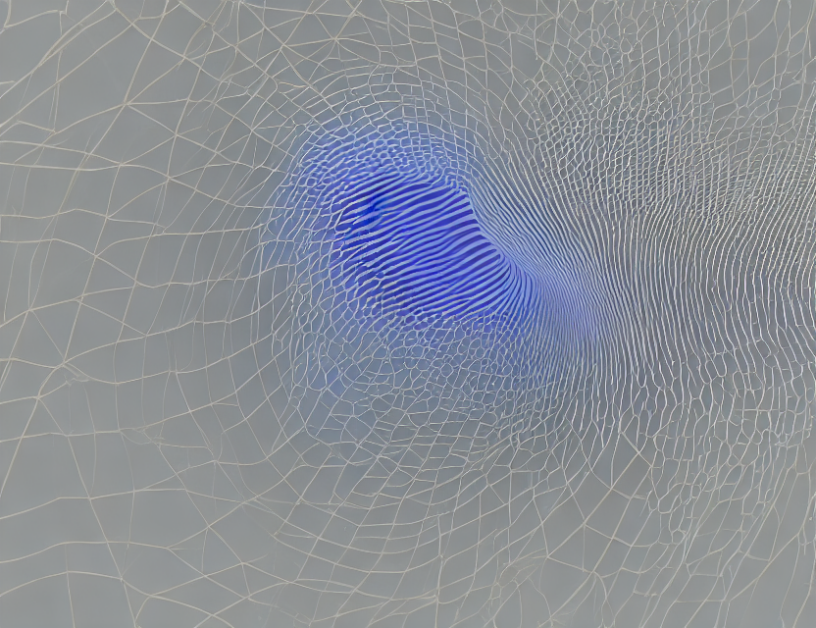
In this article, we explore the complex relationship between vortices and acoustic waves in a fluid flow. By examining the energy flux in the core to the critical layer, we discover that there is a logarithmic singularity at the point where the critical layer meets the vortex core. This singularity provides a break in the energy flux when passing through the vicinity of the critical layer. Additionally, we find that outside this vicinity, the third term in equation 21 makes a significant contribution to the energy flux.
We then examine the role of convection in the flow by considering the perturbations in the region ρ > R. Neglecting the convection of disturbances, we write down the velocity potential for a sound wave with the condition of radiation at infinity through the Hankel function. Limiting ourselves to the case of the second harmonic, m = 2, we decompose the decomposition (24) in the near region, kρ << 1, and write down the velocity potential as a sum of two terms: one representing the acoustic wave and the other, the vortex-core interaction.
Finally, we conclude that the consideration of subtle effects makes sense only for those flows that are hydrodynamically stable in the absence of these effects. The work therefore focuses on vortices with a monotonically decreasing vorticity profile, which are hydrodynamically stable. By stitching the inner solution obtained above in the incompressible fluid approximation with the outer solution in the region ρ > R, we obtain a complete picture of the flow.
Acoustic Instability of Vortices in Fluid Flows